The Hilbert space formulation of quantum mechanics is due to von Neumann(1903-1957). I cannot emphasize too much the importance of his work. In this section, we introduce the mathematical results concerning the Hilbert space without the proofs.

2.1.1:Hilbert space and operator algebra

Let ${\mathbb C}$ be the set of all complex numbers. Let $H$ be a complex Hilbert space with a inner product $\langle \cdot , \cdot \rangle $, where the inner product $\langle \cdot , \cdot \rangle : H \times H \to {\mathbb C} $ satisfies that

$\bullet$ ${\langle w, w \rangle} \ge 0 \; (\forall w \in H )$, $\qquad$ $\quad$
$\bullet$${\langle w, w \rangle}=0 \Leftrightarrow w=0$,
$\bullet$${\langle w, \alpha_1 w_1 + \alpha_2 w_2 \rangle} = \alpha_1 {\langle w, w_1 \rangle}+ \alpha_2 {\langle w, w_2 \rangle} \quad (\forall w, w_1, w_2 \in H, \forall \alpha_1, \alpha_2 \in {\mathbb C})$,
$\bullet$${\langle w_1, w_2 \rangle} = \overline{\langle w_2, w_1 \rangle}$ (i.e.,， conjugate complex) $(\forall w_1, w_2 \in H)$

And, defining the norm $\| u \| $ (or $\|u \|_H $) by $\| u \| =| \langle u , u \rangle|^{1/2} $, we get the Banach space $(H, \| \cdot \|)$. Define $B(H)$ by

\begin{align} B(H)=\{T:H \to H \;| \; T \mbox{ is a continuous linear operator} \} \tag{2.1} \end{align}

$B(H)$ is regarded as the Banach space with the operator norm $\| \cdot \|_{B(H)}$,

where \begin{align} \| T \|_{B(H)} = \sup_{\| x \|_H =1} \|T x \|_H \quad ( \forall T \in B(H) ) \tag{2.2} \end{align}

Let $T \in B(H)$. The dual operator $T^* \in B(H)$ of $T$ is defined by

\begin{align*} \langle T^* u, v \rangle = \langle u, T v \rangle \quad (\forall u,v \in H) \end{align*} The followings are clear. \begin{align*} (T^*)^* =T, \quad (T_1 T_2 )^* = T_2^* T_1^* \end{align*}

Furthermore, the following equality (called the "$C^*$-condition" ) holds:

\begin{align} \|T^* T\|=\|T T^* \|=\|T\|^2=\|T^* \|^2 \quad (\forall T \in B(H)) \tag{2.3} \end{align}

When $T=T^*$ holds, $T$ is called a self-adjoint operator (or, Hermitian operator). Let $T_n (n \in {\mathbb N} =\{ 1,2, \cdots\} ),T \in B(H)$. The sequence $\{T_n \}_{n=1}^\infty $ is said to converge in the sense of the (operator) norm topology to $T$ (that is, $n-\lim_{n \to \infty } T_n = T$), if

\begin{align*} \lim_{n \to \infty} || T_n - T ||_{B(H)}=0 \end{align*}

Also, the sequence $\{T_n \}_{n=1}^\infty $ is said to converge weakly to $T$ (that is, $w-\lim_{n \to \infty } T_n = T$ ), if

\begin{align} \lim_{n \to \infty} \langle u, (T_n - T) u \rangle = 0 \qquad (\forall u \in H ) \tag{2.4} \end{align}

Thus, we have two convergences (i.e., norm convergence and weakly convergence) in $B(H)$.


Definition 2.1 [$C^*$-algebra and $W^*$-algebra]
${\mathcal A} (\subseteq B(H) )$ is called a $C^*$-algebra, if it satisfies that
$(A_1):$${\mathcal A} (\subseteq B(H) )$ is the closed linear space in the sense of the operator norm $\| \cdot \|_{B(H)}$.
$(A_2):$${\mathcal A}$ is $\ast$-algebra, that is, ${\mathcal A} (\subseteq B(H) )$ satisfies that \begin{align*} F_1, F_2 \in {\mathcal A} \Rightarrow F_1 \cdot F_2 \in {\mathcal A}, \qquad F \in {\mathcal A} \Rightarrow F^* \in {\mathcal A} \end{align*}
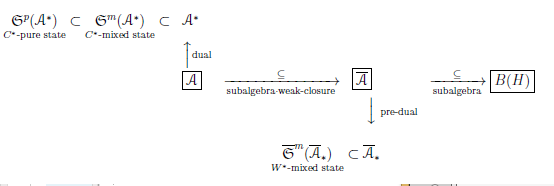
Also, a $C^*$-algebra${\mathcal A} (\subseteq B(H) )$ is called a $W^*$-algebra, if it is weak closed in $B(H)$.

2.1.2 Basic structure$[{\mathcal A} \subseteq \overline{\mathcal A} \subseteq B(H)]$;$\;\;$ general theory

Definition 2.2. [General basic structure:$[{\mathcal A} \subseteq \overline{\mathcal A} \subseteq B(H)]$]
Consider the basic structure $[{\mathcal A} \subseteq \overline{\mathcal A} \subseteq B(H)]$ $\Big($ or, denoted by $[{\mathcal A} \subseteq \overline{\mathcal A}]_{ B(H)}$ $\Big)$. That is, (A): ${\mathcal A} ( \subseteq B(H))$ is a $C^*$-algebra, and $\overline{\mathcal A} ( \subseteq B(H))$ is the weak closure of ${\mathcal A}$. Note that $W^*$-algebra $\overline{\mathcal A}$ has the pre-dual Banach space $\overline{\mathcal A}_*$( that is, $(\overline{\mathcal A}_*)^*=\overline{\mathcal A}$) uniquely. Therefore, the basic structure$[{\mathcal A} \subseteq \overline{\mathcal A} \subseteq B(H)]$ is represented as follows.
General basic structure:$[{\mathcal A} \subseteq \overline{\mathcal A} \subseteq B(H)]$

2.1.3: Basic structure$[{\mathcal A} \subseteq \overline{\mathcal A} \subseteq B(H)]$ and state space;$\;\;$General theory

The concept of "state space" is fundamental in quantum language. This is formulated in the dual space ${\mathcal A}^*$ of $C^*$-algebra ${\mathcal A}$ (or, in the pre-dual space $\overline{\mathcal A}_*$ of $W^*$-algebra $\overline{\mathcal A}$).

Let us explain it as follows.

Definition 2.3.[State space, mixed state space]
Consider the basic structure: \begin{align*} [{\mathcal A} \subseteq \overline{\mathcal A} \subseteq B(H)] \end{align*} Let ${\mathcal A}^*$ be the dual space of the $C^*$-algebra${\mathcal A}$. The mixed state space ${\frak S}^m({\mathcal A}^*)$ and the {pure state space ${\frak S}^p({\mathcal A}^*)$ is respectively defined by
(a):${\frak S}^m({\mathcal A}^*)=\{ \rho \in {\mathcal A}^* \;| \; \|\rho \|_{{\mathcal A}^*}=1, \rho \ge 0 \mbox{ (i.e., $\rho(T^* T) \ge 0 (\forall T \in {\mathcal A})$)}\}$
(b):${\frak S}^p({\mathcal A}^*)=\{ \rho \in {\frak S}^m({\mathcal A}^*) \; | \; \rho \mbox{ is a pure state} \}$. Here, $\rho (\in {\frak S}^m({\mathcal A}^*))$ is a pure state if and only if \begin{align*} \rho = \alpha \rho_1 + (1-\alpha ) \rho_2, \;\; \rho_1 , \rho_2 \in {\frak S}^m({\mathcal A}^*), 0 < \alpha <1 \Longrightarrow \rho=\rho_1=\rho_2 \end{align*}
The mixed state space ${\frak S}^m({\mathcal A}^*)$ and the pure state space ${\frak S}^p({\mathcal A}^*)$ are　locally compact spaces

Assume that $\overline{\mathcal A}_*$ is the pre-dual space of $\overline{\mathcal A}$. Then, another mixed state space $\overline{\frak S}^m(\overline{\mathcal A}_*)$　is defined by

(c):$\overline{\frak S}^m({\overline{\mathcal A}}_*) =\{ \rho \in {\overline{\mathcal A}}_* \;| \; \|\rho \|_{\overline{\mathcal A}_*}=1, \rho \ge 0 \mbox{ (i.e., $\rho(T^* T) \ge 0 (\forall T \in \overline{\mathcal A})$)}\}$

That is,　we have two "mixed state spaces",　that is,　$C^*$-mixed state space ${\frak S}^m({\mathcal A}^*)$ and　$W^*$-mixed state space $\overline{\frak S}^m({\overline{\mathcal A}}_*)$.

The above arguments are summarized in the following figure:
General basic structure:$[{\mathcal A} \subseteq \overline{\mathcal A} \subseteq B(H)]$ and states
Remark2.4　　In order to avoid the confusions,　three "state spaces" should be explained in what follows.
(D): $ \left\{\begin{array}{ll} \mbox{Fisher statistics} & \cdots \mbox{pure state space:}{{\frak S}^p({\mathcal A}^*)} \color{blue}{\mbox{: most fundamental }} \\ \\ \mbox{Bayes statistics} & \cdots \left\{\begin{array}{ll} \mbox{$C^*$-mixed state space:${{\frak S}^m({\mathcal A}^*)}$} \color{blue}{\mbox{: easy }} \\ \\ \mbox{$W^*$-mixed state space:${\overline{\frak S}^m(\overline{\mathcal A}_*)}$} \color{blue}{\mbox{: natural, useful }} \end{array}\right. \end{array}\right. $
In this note,　we mainly discuss Fisher statistics　(i.e., $\mbox{pure state space:}{{\frak S}^p({\mathcal A}^*)}$). In the case case of Bayesian statistics,　we chiefly devote ourselves to　the $W^*$-mixed state${\overline{\frak S}^m(\overline{\mathcal A}_*)}$　rather than　the　$C^*$-mixed state} ${{\frak S}^m({\mathcal A}^*)}$,　though the two play the similar roles in quantum language.