6.3(1): Population mean (Confidence interval )

Consider the classical basic structure: \begin{align} [ C_0(\Omega ) \subseteq L^\infty (\Omega, \nu ) \subseteq B(L^2 (\Omega, \nu ))] \end{align}

Fix a positive number $\alpha$ such that $0 < \alpha \ll 1$, for example, $\alpha = 0.05$.

6.3.1 Preparation (simultaneous normal measurement)

Example6.7 Consider the simultaneous normal measurement ${\mathsf M}_{L^\infty ({\mathbb R} \times {\mathbb R}_+)}$ $({\mathsf O}_G^n = ({\mathbb R}^n, {\mathcal B}_{\mathbb R}^n, {{{G}}^n}) ,$ $S_{[(\mu, \sigma)]})$ in $L^\infty({\mathbb R} \times {\mathbb R}_+)$. Here, the simultaneous normal observable ${\mathsf O}_G^n = ({\mathbb R}^n, {\mathcal B}_{\mathbb R}^n, {{{G}}^n} )$ is defined by

\begin{align} & [{{{G}}}^n ({\mathop{\mbox{$\times$}}}_{k=1}^n \Xi_k)] (\omega) = {\mathop{\mbox{ $\times$}}}_{k=1}^n [{{{G}}}(\Xi_k)](\omega) \nonumber \\ = & \frac{1}{({{\sqrt{2 \pi }\sigma{}}})^n} \underset{{{\mathop{\mbox{$\times$}}}_{k=1}^n \Xi_k }}{\int \cdots \int} \exp[{}- \frac{\sum_{k=1}^n ({}{x_k} - {}{\mu} )^2 } {2 \sigma^2} {}] d {}{x_1} d {}{x_2}\cdots dx_n \tag{6.24} \\ & \qquad (\forall \Xi_k \in {\cal B}_{{\mathbb R}{}}^{} (k=1,2,\ldots, n), \quad \forall {}{\omega}=(\mu, \sigma ) \in \Omega = {\mathbb R}\times {\mathbb R}_+). \nonumber \end{align} Therefore,the state space $\Omega$ and the measured value space $X$ are defined by \begin{align} & \Omega = {\mathbb R} \times {\mathbb R}_+ \\ & X={\mathbb R}^n \end{align} Also, the second state space $\Theta$ is defined by \begin{align} & \Theta = {\mathbb R} \end{align}

The estimator $E: {\mathbb R}^n \to \Theta (\equiv {\mathbb R} )$ and the system quantity$\pi: \Omega \to \Theta $ are respectively defined by

\begin{align} & E(x)=E(x_1, x_2, \ldots , x_n ) = \overline{\mu}(x) = \frac{x_1 + x_2 + \cdots + x_n}{n} \\ & \Omega={\mathbb R} \times {\mathbb R}_+ \ni \omega = (\mu, \sigma ) \mapsto \pi (\omega ) = \mu \in \Theta={\mathbb R} \end{align}

Also, the semi-metric $d_{\Theta}^{(1)}$ in $\Theta$ is defined by

\begin{align} d_{\Theta}^{(1)}(\theta_1, \theta_2) = |\theta_1 - \theta_2| \qquad (\forall \theta_1, \theta_2 \in \Theta ={\mathbb R}) \nonumber \end{align}

6.3.2 Confidence interval Our present problem is as follows.
Problem 6.8 [Confidence interval].

Consider the simultaneous normal measurement ${\mathsf M}_{L^\infty ({\mathbb R} \times {\mathbb R}_+)}$ $({\mathsf O}_G^n = ({\mathbb R}^n, {\mathcal B}_{\mathbb R}^n, {{{G}}^n}) ,$ $S_{[(\mu, \sigma)]})$. Assume that a measured value$x \in X ={\mathbb R}^n$ is obtained by the measurement. Let $0 < \alpha \ll 1$.
Then, find the ${D}_{x}^{1- \alpha; \Theta}( \subseteq \Theta)$ (which may depend on $\sigma$) such that

$\bullet:$ the probability that $\mu \in {D}_{x}^{1- \alpha; \Theta}$ is more than $1-\alpha$.
Here, the more ${D}_{x}^{1- \alpha; \Theta}( \subseteq \Theta)$ is small, the more it is desirable.

Consider the following semi-distance $d_{\Omega}^{(1)}$ in the state space ${\mathbb R} \times {\mathbb R}_+$:

\begin{align} d_{\Omega}^{(1)}((\mu_1,\sigma_1), (\mu_2,\sigma_2)) = |\mu_1 - \mu_2| \tag{6.25} \end{align}

For any $ \omega=(\mu, \sigma ) (\in\Omega= {\mathbb R} \times {\mathbb R}_+ )$, define the positive number $\delta^{1 - \alpha }_{\omega}$ $(> 0)$ such that:

\begin{align} \delta^{1 - \alpha }_{\omega} = \inf \{ \eta > 0: [F (E^{-1} ( {{ Ball}_{d_\Omega^{(1)}}}(\omega ; \eta))](\omega ) \ge {1 - \alpha } \} \nonumber \end{align}

where ${{ Ball}_{d_\Omega^{(1)}}}(\omega ; \eta)$ $=$ $\{ \omega_1 (\in\Omega): d_\Omega^{(1)} (\omega, \omega_1) \le \eta \}$ $= [\mu - \eta , \mu + \eta ] \times {\mathbb R}_+$

Hence we see that \begin{align} & E^{-1}({{ Ball}_{d_\Omega^{(1)}}}(\omega ; \eta )) = E^{-1}([\mu - \eta , \mu + \eta ] \times {\mathbb R}_+) \nonumber \\ = & \{ (x_1, \ldots , x_n ) \in {\mathbb R}^n \;: \; \mu - \eta \le \frac{x_1+\ldots + x_n }{n} \le \mu + \eta \} \tag{6.26} \end{align} Thus, \begin{align} & [{{{G}}}^n (E^{-1}({{ Ball}_{d_\Omega^{(1)}}}(\omega ; \eta ))] (\omega) \nonumber \\ = & \frac{1}{({{\sqrt{2 \pi }\sigma{}}})^n} \underset{{ \mu - \eta \le \frac{x_1+\ldots + x_n }{n} \le \mu + \eta }}{\int \cdots \int} \exp[{}- \frac{\sum_{k=1}^n ({}{x_k} - {}{\mu} )^2 } {2 \sigma^2} {}] d {}{x_1} d {}{x_2}\cdots dx_n \nonumber \\ = & \frac{1}{({{\sqrt{2 \pi }\sigma{}}})^n} \underset{{ - \eta \le \frac{x_1+\ldots + x_n }{n} \le \eta }}{\int \cdots \int} \exp[{}- \frac{\sum_{k=1}^n ({}{x_k} {}{} )^2 } {2 \sigma^2} {}] d {}{x_1} d {}{x_2}\cdots dx_n \nonumber \\ \end{align} Using the Gauss integral (6.6), we see \begin{align} = & \frac{\sqrt{n}}{{\sqrt{2 \pi }\sigma{}}} \int_{{- \eta}}^{\eta} \exp[{}- \frac{{n}{x}^2 }{2 \sigma^2}] d {x} = \frac{1}{{\sqrt{2 \pi }{}}} \int_{{- \sqrt{n} \eta/\sigma}}^{\sqrt{n} \eta / \sigma} \exp[{}- \frac{{x}^2 }{2 }] d {x} \tag{6.27} \end{align} 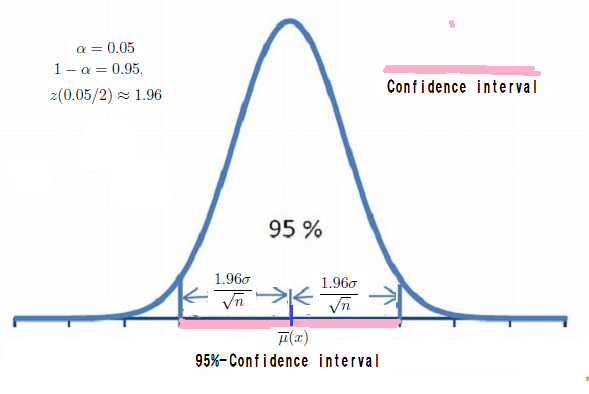Solving the following equation: \begin{align} \frac{1}{{\sqrt{2 \pi }{}}} \int^{-z({ \alpha }/2)}_{-\infty} \exp[{}- \frac{{x}^2 }{2 }] d {x} = \frac{1}{{\sqrt{2 \pi }{}}} \int_{z({ \alpha }/2)}^{\infty} \exp[{}- \frac{{x}^2 }{2 }] d {x} = \frac{\alpha}{2} \tag{6.28} \end{align} we define that \begin{align} \delta^{1 - \alpha }_{\omega} = \frac{\sigma}{\sqrt{n}} z(\frac{\alpha}{2}) \tag{6.29} \end{align}

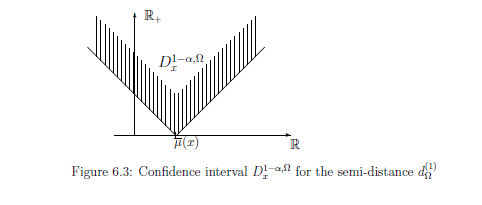
Then, for any $x$ $(\in {\mathbb R}^n)$, we get $D_x^{{1 - \alpha }, \Omega}$ ( the $({1 - \alpha })$-confidence interval of $x$ ) as follows:

\begin{align} D_x^{{1 - \alpha, \Omega }} & = \{ {\omega} (\in \Omega) : d_\Omega (E(x), \omega) \le \delta^{1 - \alpha }_{\omega } \} \nonumber \\ & = \{ (\mu, \sigma ) \in {\mathbb R} \times {\mathbb R}_+ \;:\; | \mu - \overline{\mu}(x)| = | \mu - \frac{x_1+ \ldots + x_n}{n}| \le \frac{\sigma}{\sqrt{n}} z(\frac{\alpha}{2}) \} \tag{6.30} \end{align}
Also, \begin{align} D_x^{{1 - \alpha, \Theta }} & = \{ \pi ({\omega}) (\in \Theta) : d_\Omega (E(x), \omega) \le \delta^{1 - \alpha }_{\omega } \} \nonumber \\ & = \{ \mu \in {\mathbb R} \;:\; | \mu - \overline{\mu}(x)| = | \mu - \frac{x_1+ \ldots + x_n}{n}| \le \frac{\sigma}{\sqrt{n}} z(\frac{\alpha}{2}) \} \nonumber \end{align} which depends on $\sigma$.