Definition 8.1[(=Definition 3.19Definition):quasi-product product{ observable ]

Let ${\mathsf O}_k$ ${{=}}$ $(X_k , $ ${\cal F}_k , $ $F_k)$ ($k = 1,2,\ldots, n$ ) be observables in a $W^*$-algebra $\overline{\mathcal A}$. Assume that an observable ${\mathsf O}_{12...n}$ ${{=}}$ $(\times_{k=1}^n X_k ,$ $ \boxtimes_{k=1}^n{\cal F}_k , $ ${F}_{12...n})$ satisfies

\begin{align} & {F}_{12...n}(X_1 \times \cdots \times X_{k-1} \times \Xi_k \times X_{k+1} \times \cdots \times X_n ) = F_k (\Xi_k) \tag{8.1} \\ & \quad \qquad \qquad (\forall \Xi_k \in {\cal F}_k , \forall k =1,2,\ldots,n ) \nonumber \end{align}

The observable ${\mathsf O}_{12...n}$ ${{=}}$ $(\times_{k=1}^n X_k ,$ $ \boxtimes_{k=1}^n{\cal F}_k , $ ${F}_{12...n})$ is called a quasi-product{ observable of $\{ {\mathsf O}_k \; | \;k=1,2,\ldots,n \}$, and denoted by

\begin{align} {\mathop{\overset{qp}{\times}}_{k=1,2,\ldots,n}}{\mathsf O}_k = (\times_{k=1}^n X_k , \boxtimes_{k=1}^n{\cal F}_k, {\mathop{\overset{qp}{\times}}_{k=1,2,\ldots,n} }F_k) \end{align}

Of course, a simultaneous observable is a kind of quasi-product observable. Therefore, quasi-product observable is not uniquely determined. Also, in quantum systems, the existence of the quasi-product observable is not always guaranteed.

Definition 8.2[Image observable, marginal observable] Consider the basic structure$[ {\mathcal A} \subseteq \overline{\mathcal A} \subseteq B(H)]$. And consider the observable ${\mathsf O}$ ${{=}}$ $( X,$ ${\cal F} , $ ${F})$ in $\overline{\mathcal A}$. Let $(Y, {\mathcal G})$ be a measurable space, and let $f: X \to Y $ be a measurable map. Then, we can define the {image observable $f( {\mathsf O})$ ${{=}}$ $( X,$ ${\cal F} , $ ${F}\circ f^{-1})$ in $\overline{\mathcal A}$, where ${F}\circ f^{-1}{}$ is defined by

\begin{align} ( {F}\circ f^{-1}{} ) (\Gamma ) = F( f^{-1}(\Gamma )) \qquad (\forall \Gamma \in {\mathcal G} ) \end{align}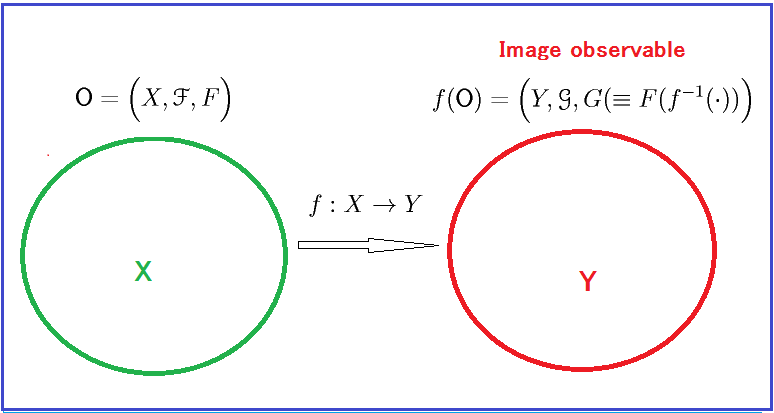

[Marginal observable ] Consider the basic structure$[ {\mathcal A} \subseteq \overline{\mathcal A} \subseteq B(H)]$. And consider the observable ${\mathsf O}_{12...n}$ ${{=}}$ $(\times_{k=1}^n X_k ,$ $ \boxtimes_{k=1}^n{\cal F}_k , $ ${F}_{12...n})$ in $\overline{\mathcal A}$. For any natural number $j$ such that $1{{\; \leqq \;}}j {{\; \leqq \;}}n$, define ${F}^{(j)}_{12...n}$ such that

\begin{align} {F}^{(j)}_{12...n} (\Xi_j) = F_{12...n}(X_1 \times \cdots \times X_{j-1} \times \Xi_j \times X_{j+1} \times \cdots \times X_{n}) \quad ( \forall \Xi_j \in {\cal F}_j ) \end{align}

Then we have the observable ${\mathsf O}^{(j)}_{12...n}$ ${{=}}$ $(X_j ,$ $ {\cal F}_j , $ ${F}^{(j)}_{12...n})$ in $\overline{\mathcal A}$. The ${\mathsf O}^{(j)}_{12...n}$ is called a marginal observable of ${\mathsf O}_{12...n}$ ( or, precisely, $(j)$-marginal observable ). Consider a map $P_j: \times_{k=1}^n X_k \to X_j$ such that

\begin{align} \times_{k=1}^n \ni (x_1, x_2, ..., x_j, ...,x_n ) \mapsto x_j \in X_j \end{align}

Then, the marginal observable ${\mathsf O}^{(j)}_{12...n}$ is characterized as the image observable $P_j( {\mathsf O}_{12...n} )$.


The above can be easily generalized as follows. For example, define ${\mathsf O}_{12...n}^{(12)}$ ${{=}}$ $( X_1 \times X_2 ,$ $ {\cal F}_1 \boxtimes {\cal F}_2, $ ${F}^{(12)}_{12...n})$ such that

\begin{align} {F}^{(12)}_{12...n}(\Xi_1 \times \Xi_2) = F^{(12)}_{12...n}(\Xi_1 \times \Xi_2 \times X_3 \times \cdots \times X_{n}) \quad ( \forall \Xi_1 \in {\cal F}_1, \forall \Xi_2 \in {\cal F}_2 ) \end{align}

Then, we have the $(12)$-marginal observable ${\mathsf O}_{12...n}^{(12)}$ ${{=}}$ $( X_1 \times X_2 ,$ $ {\cal F}_1 \boxtimes {\cal F}_2, $ ${F}^{(12)}_{12...n})$. Of course, we also see that ${F}_{12...n}={F}^{(12...n)}_{12...n}{}$.


The following theorem is often used:
Theorem 8.3 Consider the basic structure \begin{align} [ {\mathcal A} \subseteq \overline{\mathcal A} \subseteq B(H)] \end{align}

Let $\overline{\cal A }$ be a $C^*$-algebra. Let ${\mathsf O}_1$ $\equiv$ $(X_1 , {\cal F}_1 , F_1 ) $ and ${\mathsf O}_2$ $\equiv$ $(X_2 , {\cal F}_2 , F_2 ) $ be $W^*$-observables in $\overline{\cal A}$ such that at least one of them is a projective observable. $\Big($So, without loss of generality, we assume that ${\mathsf O}_2$ s projective, i.e., $F_2 (F_2)^2$ $\Big)$. $\; \; $ Then, the following statements are equivalent:

$(i):$There exists a quasi-product observable\ ${\mathsf O}_{12}$ $\equiv$ $(X_1 \times X_2 , {\cal F}_1 \times {\cal F}_2 , F_1 \overset{qp}{\times} F_2)$ with marginal observables ${\mathsf O}_1$ and ${\mathsf O}_2$.
(ii):${\mathsf O}_1$ and ${\mathsf O}_2$ commute, that is, $ F_1 (\Xi_1 ) F_2 (\Xi_2 ) $ $ =$ $ F_2 (\Xi_2 )F_1 (\Xi_1 ) $ $(\forall \Xi_1 \in {\cal F}_1 , \forall \Xi_2 \in {\cal F}_2 ) $.

Furthermore, if the above statements (i) and (ii) hold, the uniqueness of the quasi-product observable ${\mathsf O}_{12}$ of ${\mathsf O}_{1}$ and ${\mathsf O}_{2}$ is guaranteed.